√ダウンロード q table tukey 0.05 552573

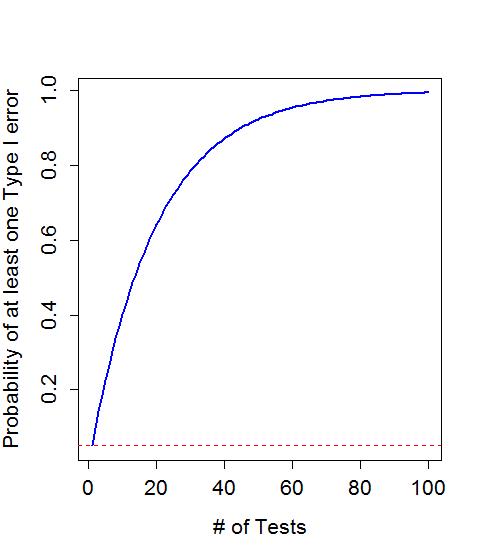
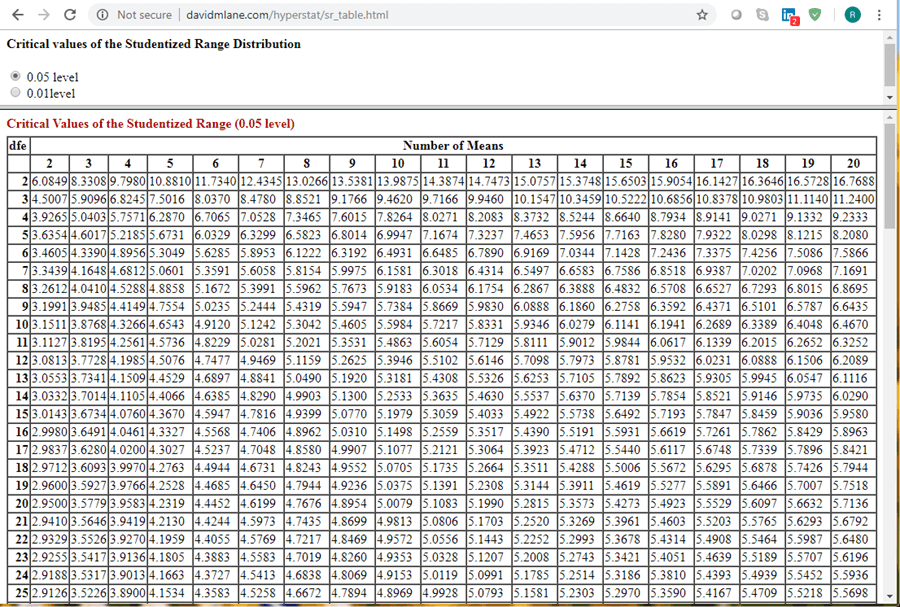
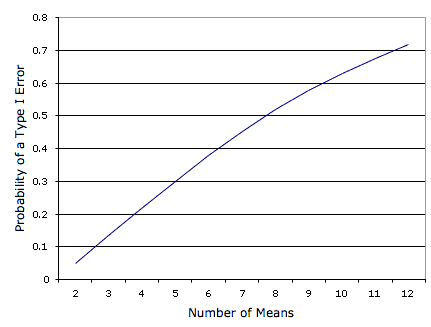
764 0 732 141Dashed line indicates 005 In pairwise comparisons between all the pairs of means in a OneWay ANOVA, the number of tests is based on the number of pairs We can calculate the number of tests using J choose 2, ( J 2 ), to get the number of pairs of size 2 that we can make out of J individual treatment levelsThe Studentized range upper quantiles q(k, df;

Studentized Range Q Table Real Statistics Using Excel

Q table tukey 0.05

Q table tukey 0.05- A 15 2 7 B 15 2 7 C 15 0 6 alpha 005 ;005) df k> 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19

T U K E Y Q T A B L E Zonealarm Results

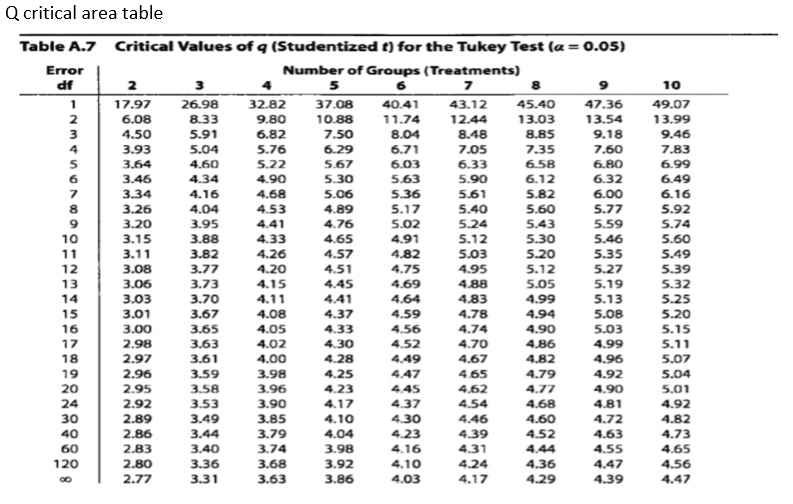
Critical Values of Studentized Range Distribution(q) for Familywise ALPHA =05Your q score goes in the first box The number of groups (or treatments, means, etc) in your study goes into the second box And you add the withingroups degrees of freedom to the final box After that, just select your significance level (it defaults to05), and hit the Calculate buttonTukey's posthoc test is a method that is used to determine which groups among the sample have significant differences This method calculates the difference between the means of all the groups Tukey's HSD test values are number which acts as a distance between the groups It works by defining a value known as Honest Significant Difference

STATISTICAL TABLES 1 TABLE A1 Cumulative Standardized Normal Distribution A(z) is the integral of the standardized normal distribution from −∞to z (in other words, the area under the curve to the left of z) It gives the probability of a normal random variable not being more than z standard deviations above its meanTukey Q Calculator This tool will calculate critical values (Q05 and Q01) for the Studentized range distribution statistic (Q), normally used in the calculation of Tukey's HSD The calculator is easy to use Just input the number of groups in your study (k) in the first box, and degrees of freedom (normally the total number of subjects minus the number of groups) in the second boxJohn Tukey made a system for managing multiple comparisons in ANOVA that still keeps the experimentwide error at or below set significance level (eg 005) without maintaining as much power as possible

The bottom (bold) value is α =01 The number of treatments is listed across The df for the error term is in the left column, where the "error term" is another name for the withintreatments variance Now, use the q value to calculate Tukey's HSD The widespread use of 'statistical significance' as a license for making a claim of a scientific finding leads to considerable distortion of the scientific process (according to the American Statistical Association) We review why degrading pvalues into 'significant' and 'nonsignificant' contributes to making studies irreproducible, or to making them seem• Untuk mencari nilai q005(6, 24) kita dapat melihatnya pada tabel Sebaran studentized range pada taraf nyata α = 005 dengan p = 6 dan derajat bebas (v)= 24 Perhatikan gambar berikut untuk menentukan qtabel • • Dari tabel tersebut kita dapatkan nilai nilai q005(6, 24) = 437 • Hitung nilai HSD dengan menggunakan formula berikut •

An Introduction To Q Learning Reinforcement Learning

Tukey Test Table Statistics

Q Distribution Table How to Use the Q Distribution Table This table should be used only if the sample sizes in your Tukey's HSD analysis are equal There are two sections ofTo evaluate Tukey's HSD, consult the _____ table q dividing 005 by the number of comparisons you plan to make and using the result as the p level for each comparison Lucille is interested in the effects of alcohol consumption on style of play while playing the videogame Sims She asks a group of 15 college students to play Sims underTukeyTable Table Q scores for Tukeys method = 005 k df 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 24 30 40 60 1 2 180 608 450 393 364 Course Hero TukeyTable Table Q scores for Tukeys method = 005 k df 1

108 Questions With Answers In Tukey Science Topic

Http Www Iceaaonline Com Ready Wp Content Uploads 16 10 Ra02 Paper Jones Outing Outliers Pdf

 Table Q scores for Tukey's method α = 005 k df 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 24 30 40 60 1 ∞ α = 001 2 3 4 5 6 7 8 9 10 180 608 45Tukey's range test, also known as Tukey's test, Tukey method, Tukey's honest significance test, or Tukey's HSD (honestly significant difference) test, is a singlestep multiple comparison procedure and statistical testIt can be used to find means that are significantly different from each other Named after John Tukey, it compares all possible pairs of means, and is based on aDf Error 28 Critical Value of Studentized Range Honestly Significant Difference Means with the same letter are not significantly different

Www Jstatsoft Org Article View V085c01 V85c01 Pdf

T U K E Y Q T A B L E Zonealarm Results

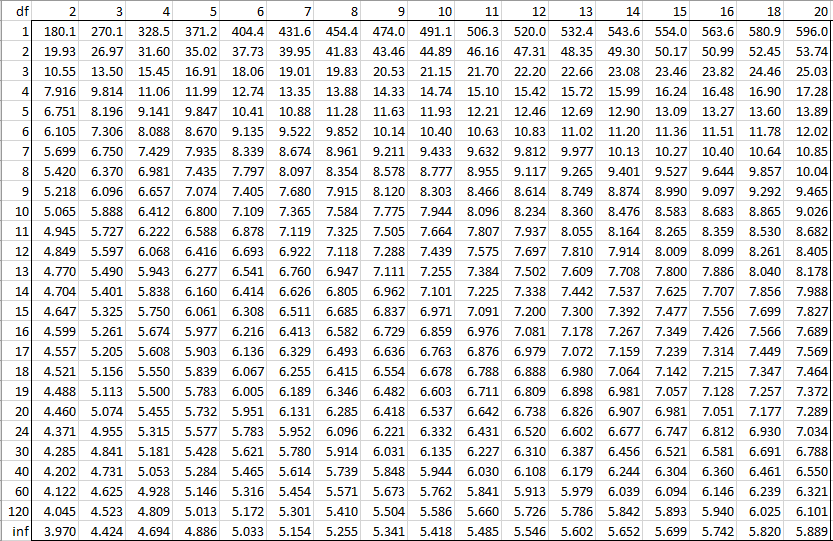
Table Q scores for Tukey's method α = 005 α = 001 k 2 3 4 5 6 7 8 9 10 k 2 3 4 5 6 7 8 9 10 df df 1 180 270 328 371 404 431 454 474 491 1 900 135 164 186 2 216 227 237 246 2 608 3 980 10 1173 1243 1303 1354 1399 2 1390 1902 2256 2537 2776 2986 3173 3341 3493 3 450 591 6 750 804 848 5 918 946 3 6 1062 1217 1332 1424 1500Statistical Table G TukeyKramer critical values Statistical Table H Critical Values for the Spearman's correlation Statistical Tables 2 denominator, respectively, for a onetailed test with α = 005 The table gives a column for df = 1 in the numerator, but skips from df = 100 to df = 0 for the denominator We Qcrit for df = 1 is 3685 and the Qcrit for df = 240 id 3659 A linear interpolation would give the value , which can be calculated using the Real Statistics formula =QCRIT(4,156,005,2) The Real Statistics formula =QINV(005,4,156,2), which does not use the table, will usually give a more accurate answer, which in this case is

Multiple Pair Wise Comparisons Using Tukey S Hsd And The Compact Letter Display Statistics With R

The Tukey Procedure With Anova Test Youtube

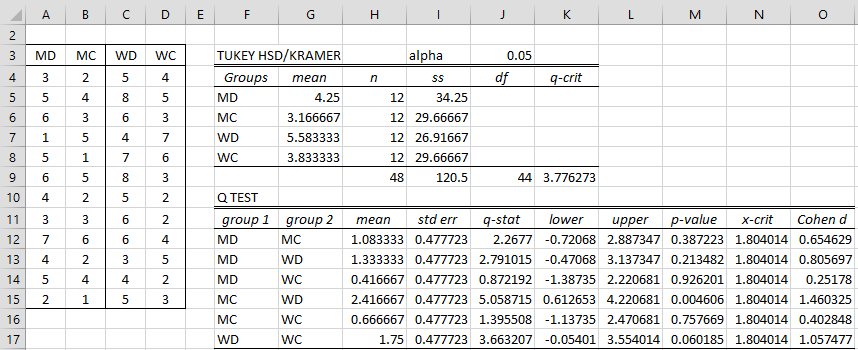
 The pvalue from the ANOVA table is Since this pvalue is less than 05, we can reject the null hypothesis and conclude that the means between the three groups are not equal To determine exactly which group means are different, we can perform a TukeyKramer post hoc test using the following stepsUsing the Studentized Range q Table with α =05, k = 4 and dfW = 44, we get qcrit = Note that since there is no table entry for df = 44, we need to interpolate between the entries for df = 40 and df = 48 Alternatively, we can employ Excel's table lookup capabilities My question is how can we obtain a Tukey upper quantile table(for studentized range distribution) table using qtukey function?(The table is like Tukey Table) (dfrange=10,nurange=,alpha=005,digs=3){ ROWS

Www Stat Purdue Edu Xuanyaoh Stat350 Xymar23lec22 Pdf

10 Tukey S Hsd Test Sleep Apnea Is A Disorder Chegg Com

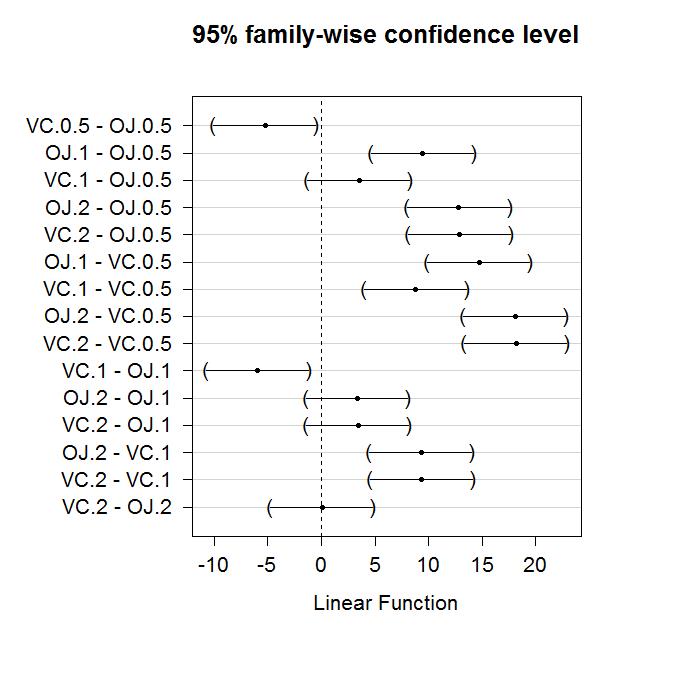
 # Tukey Honestly Signficant Difference tukeyhsd qvalue * sqrt(mse / n) tukeyhsd ## 1 As mentioned earlier, the Honestly Significant Difference is a statistic that can be used to determine significant differences between groupsComparison of 95% confidence intervals to the wider 9935% confidence intervals used by Tukey's in the previous example The reference line at 0 shows how the wider Tukey confidence intervals can change your conclusions Confidence intervals that contain zero indicate no difference (Only 5 of the 10 comparisons are shown due to spaceThus, again for an α = 005 test all we need to look at is the tdistribution for \(\alpha / 2g = \) and N a =30 df Looking at the t table we get the value 303 However, to compare with the Tukey Studentized Range statistic, we need to multiply the tabled critical value by \(\sqrt{2} = 1414\), therefore 303 x1414 = 428 , which

Graphpad Prism 9 Statistics Guide Example Planned Comparisons

T U K E Y Q T A B L E Zonealarm Results

The multiple comparison method Choose the comparison procedure based on the group means that you want to compare, the type of confidence level that you want to specify, and how conservative you want the results to be "Conservative" in this context indicates that the true confidence level is likely to be greater than the confidence level thatTable A8 Critical values (at 5% and 1% significance levels) for Duncan's Multiple Range Test α = 05 Number of consecutive means ( p ) to be comparedThe table below reports the aforementioned ANOVA omnibus test Our null hypothesis is that the population means are equal for all medicines administered P ("Sig") = 0000 way less than 005 so we reject this hypothesis the population means are not all equal Some medicines result in lower mean BDI scores than other medicines

All Pairwise Comparisons Among Means

Post Hoc Tests For One Way Anova

INTERPRETING THE ONEWAY ANOVA PAGE 2 The third table from the ANOVA output, (ANOVA) is the key table because it shows whether the overall F ratio for the ANOVA is significant Note that our F ratio (6414) is significant (p = 001) at the 05 alpha level When reporting this finding – we would write, for example, F(3, 36) = 641, p < 01 The F indicates that we are using an F test (ieQTable Numbers in the far left column are degrees of freedom Numbers in the second column are significance levels (alpha) Blue numbers in the top row refer to the number of groups df24 rows TABLE B5 The studentized range statistic (q)* *The critical values for q corresponding to alpha = 05 (top) and alpha =01 (bottom)

Studentized Range Q Table Real Statistics Using Excel

One Way Anova Independent Samples Ii

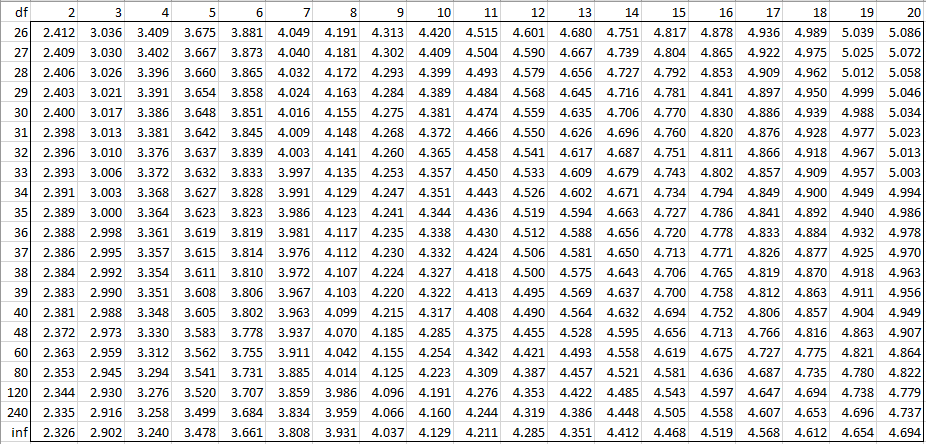
The Studentized range upper quantiles q(k,df;005) df 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 1 370Critical Values of Q Calculator Critical values of the studentized range distribution (Q) are commonly used in Tukey's range test A continuous probability distribution that arises during the estimation of the range of a normally distributed population in circumstances where the population SD is unknown and the size of the sample is also less is called as the Studentized rangeTukey's method considers all possible pairwise differences of means at the same time The Tukey method applies simultaneously to the set of all pairwise comparisons $$ \{ \mu_i \mu_j \} \, $$ The confidence coefficient for the set, when all sample sizes are equal, is exactly \(1 \alpha\) For unequal sample sizes, the confidence coefficient is greater than \(1 \alpha\)

Ch12 1 Exercises One Way Analysis Of Variance Anova

Multiple Pair Wise Comparisons Using Tukey S Hsd And The Compact Letter Display Statistics With R

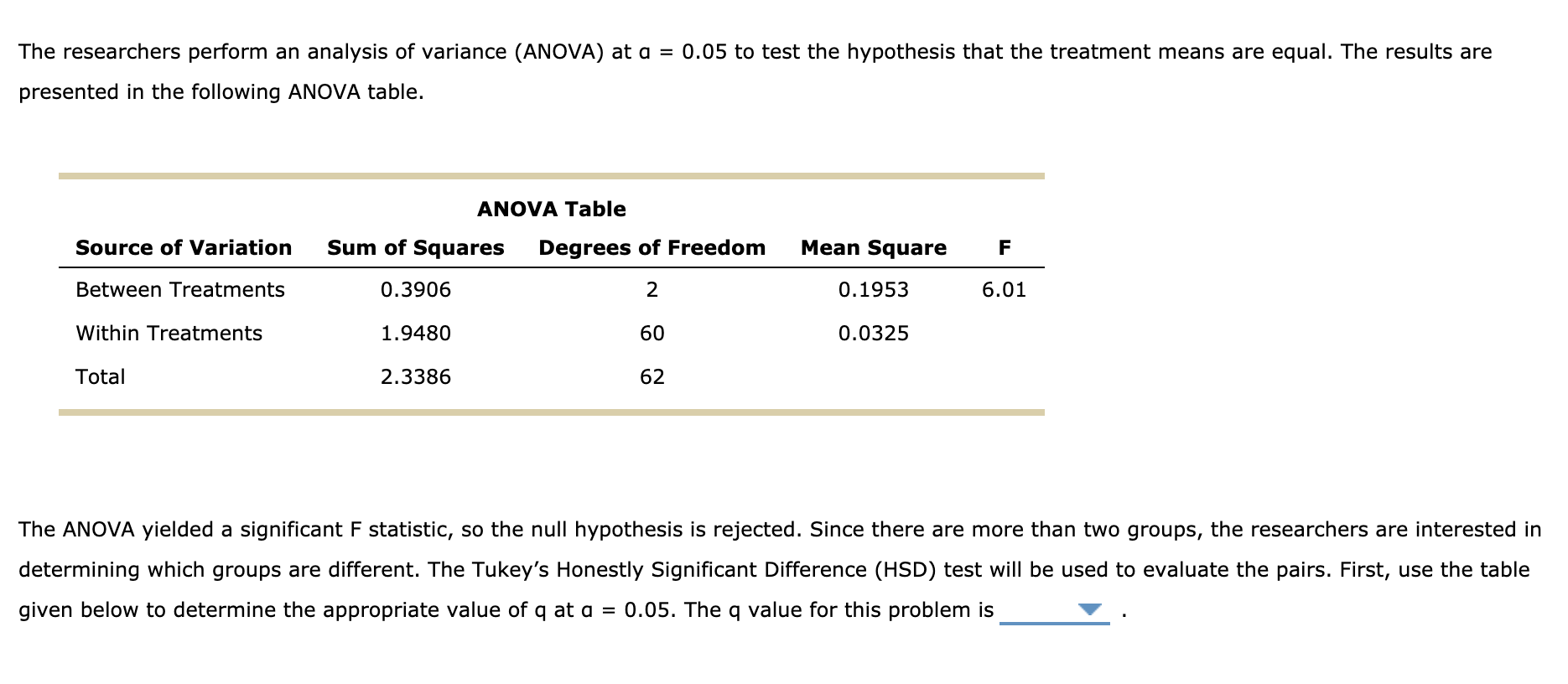
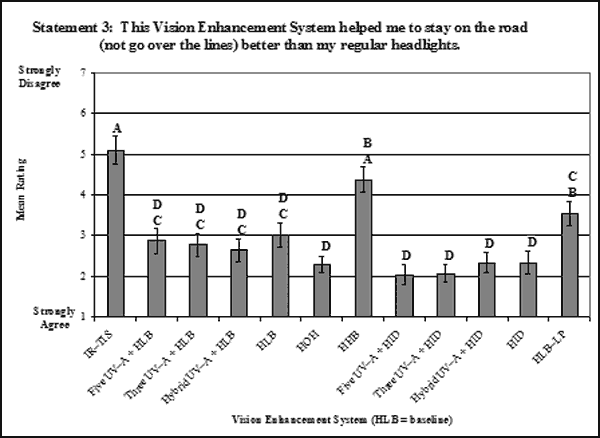
The top value is α =05;The Tukey's Honestly Significant Difference (HSD) test will be used to evaluate the pairs First, use the table at the right to determine the appropriate value of q at alpha = 05 The q value for this problem is _____ Now, use the q value to calculate Tukey's HSD Tukey's HSD is _____ If oneway ANOVA reports a P value of

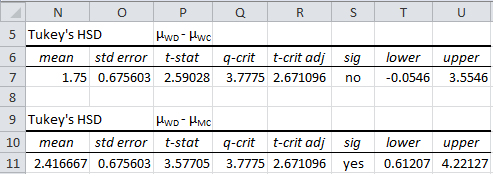
Tukey Hsd Real Statistics Using Excel

Tukey Kramer Multiple Comparisons Test If The Value Of Q Is Greater Download Table

E&CE 411, Spring 09, Table of Q Function 1 Table 1 Values of Q(x) for 0 ≤ x ≤ 9 x Q(x) x Q(x) x Q(x) x Q(x) 000 05 230 455 263×10−6 680 5231×10−12 005 235 460 ×10−6 685 ×10−12 010 240 465 ×10−6 690 ×10−12 015 245 470 ×10−6 695 164×10−12The Newman–Keuls or Student–Newman–Keuls (SNK) method is a stepwise multiple comparisons procedure used to identify sample means that are significantly different from each other It was named after Student (1927), D Newman, and M Keuls This procedure is often used as a posthoc test whenever a significant difference between three or more sample means has been revealed

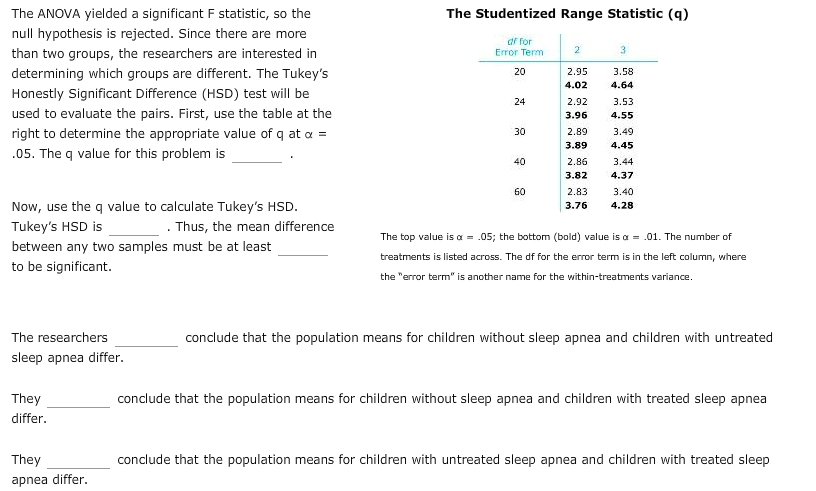
10 Tukey S Hsd Test Sleep Apnea Is A Disorder Chegg Com

R Handbook Least Square Means For Multiple Comparisons

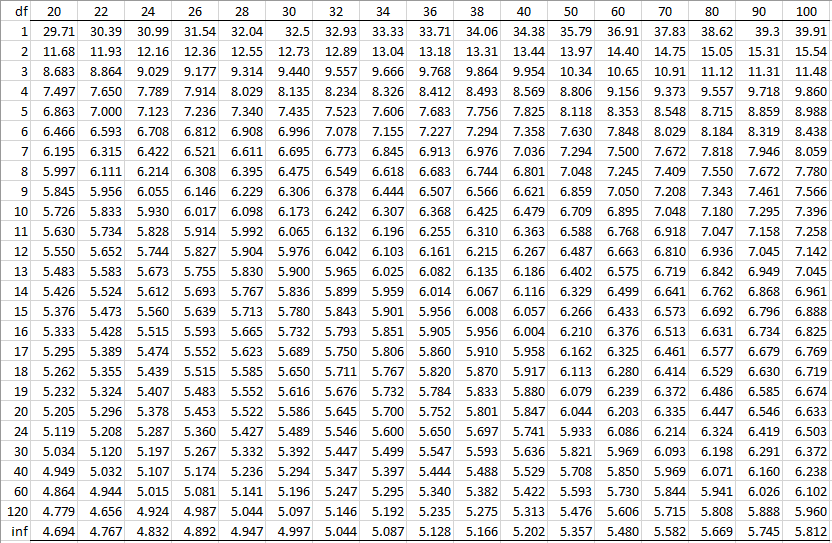
Studentized Range Q Table Real Statistics Using Excel

Q Table For Tukey Pdf Table B 5 The Q Statistic Tukey Hsd Test K Number Of Treatments Levels Withingroups Df Alp Ha Lev El 2 3 4 5 6 7 8 9 10 11 12 Course Hero

T U K E Y Q T A B L E Zonealarm Results

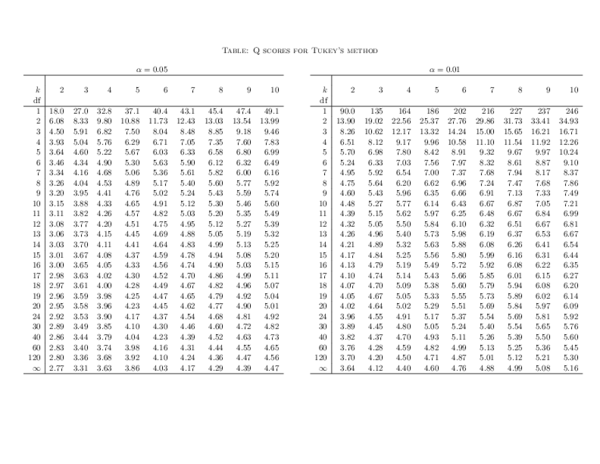
Pdf Table Q Tukey Dhal Geun Ahn Academia Edu

Magnetic Bead Based Solution For Dna Extraction From Environmental Samples Using Mag Bind Environmental Dna 96 Kit From Omega Bio Tek Omega Bio Tek

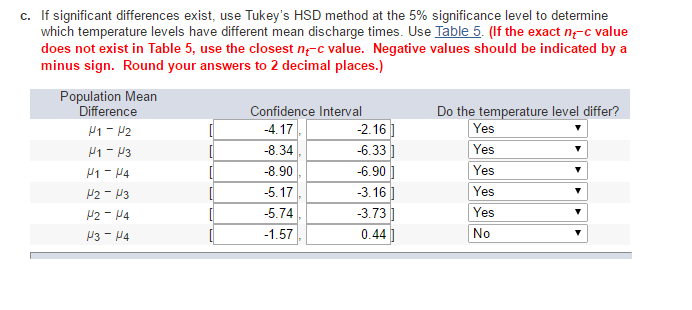
Table 5 Studentized Range Values Q For Tukey S Hsd Chegg Com

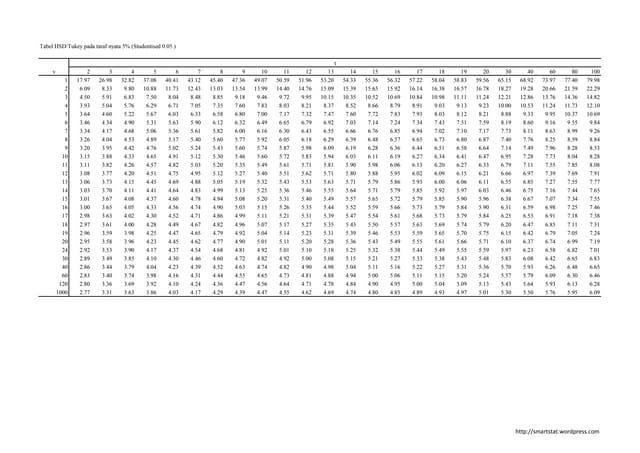
T U K E Y Q T A B L E Zonealarm Results

Www Ars Usda Gov Arsuserfiles Rapidcalculationofq Pdf

Post Hoc Tests For One Way Anova

Www Jstatsoft Org Article View V085c01 V85c01 Pdf

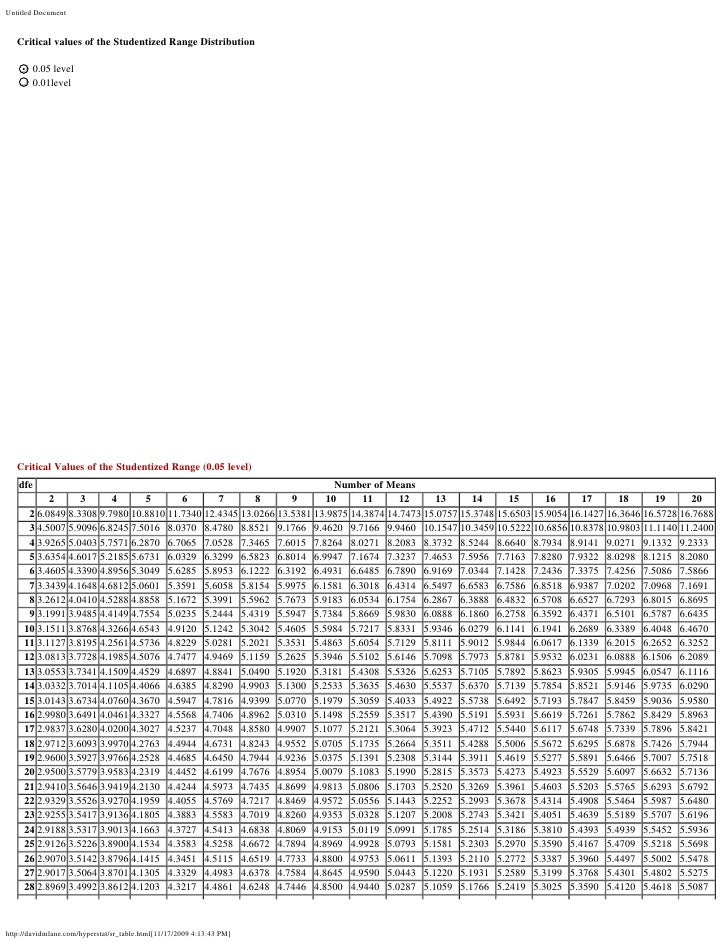
43 Q Distribution Table For Tukey Test

Rpubs Com ronsc32 Post Hoc Analysis Tukey

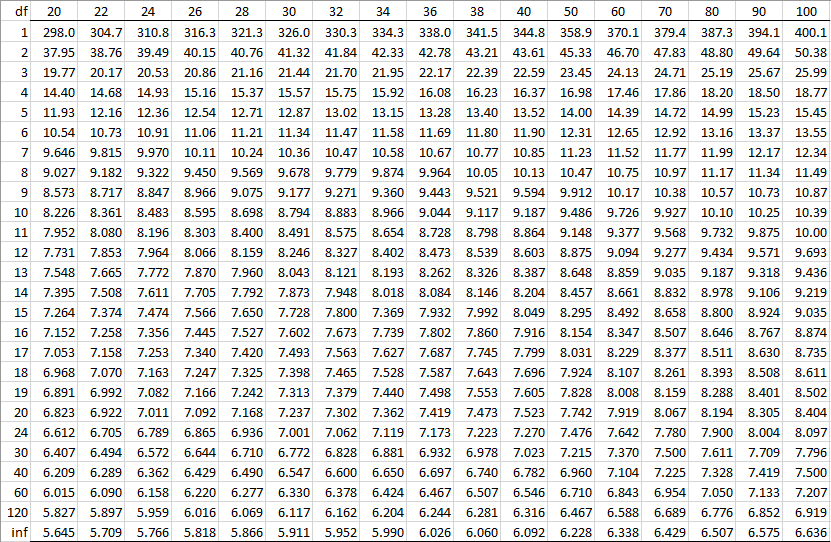
Studentized Range Q Table Real Statistics Using Excel

Multiple Comparisons Matlab Simulink

Analysis Of Variance Anova Quantitative Methods In Hpels 440 Ppt Download

T U K E Y Q T A B L E Zonealarm Results

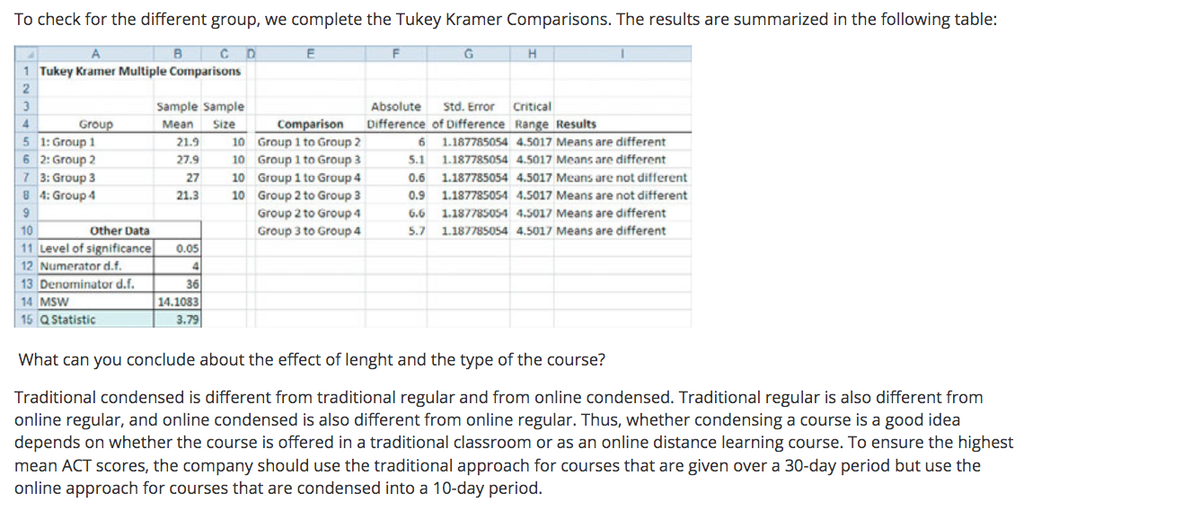
Answered To Check For The Different Group We Bartleby

Http Imathesis Com Media Tukey Crit Values Pdf

T U K E Y Q T A B L E Zonealarm Results

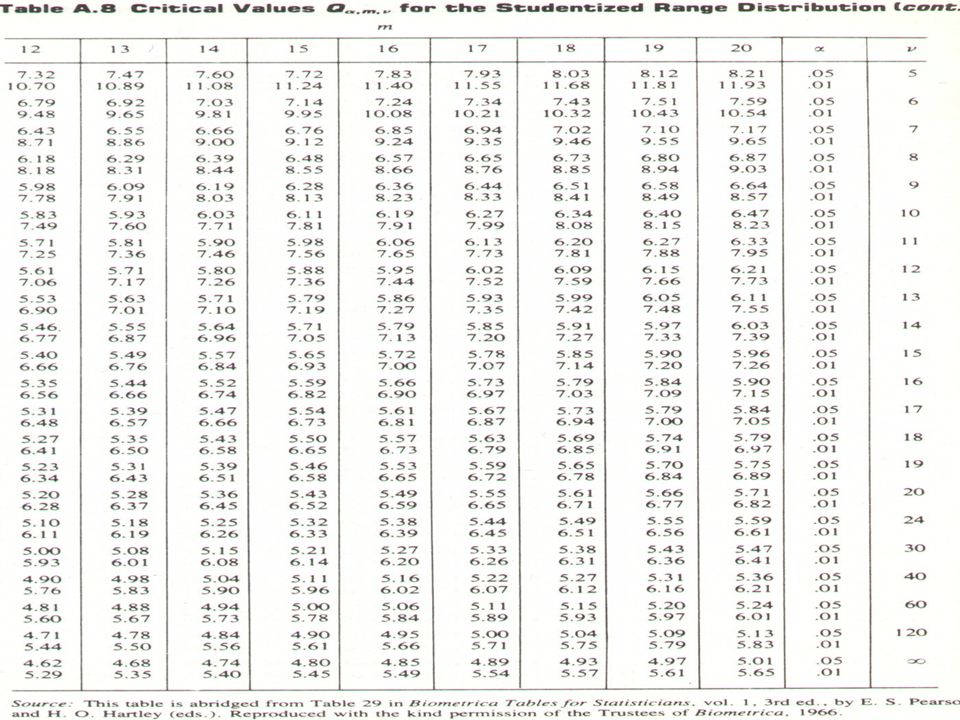
Confidence Intervals Ppt Video Online Download

How To Obtain Tukey Table In R Cross Validated

Tukeytable Table Q Scores For Tukeys Method 0 05 K Df 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 24 30 40 60 1 2 18 0 6 08 4 50 3 93 3 64 Course Hero

1 A Psychologist Would Like To Examine The Relative Effectiveness Of 3 Treatments For Mild Phobias A Sample Of N 12 Individuals Who Display A Moderate Fear Of Spiders Is Obtained These Individuals Homeworklib

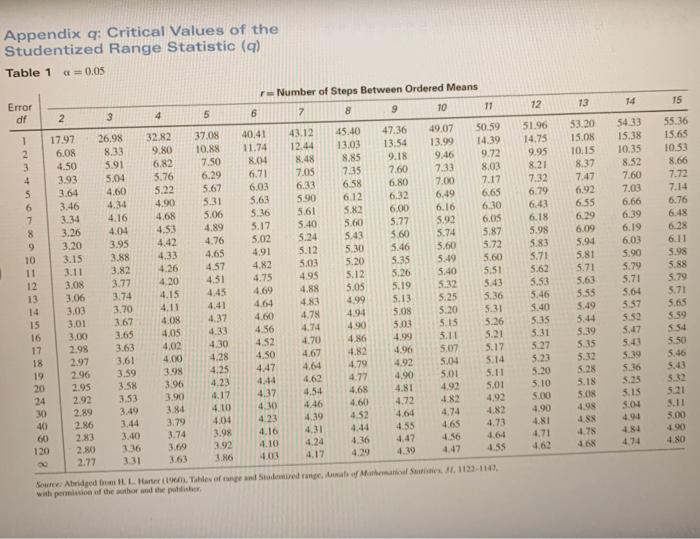
If Conducting Multiple Comparisons Using The Tukey Chegg Com

Uggsjrkh6 Ivum

Www Ars Usda Gov Arsuserfiles Rapidcalculationofq Pdf

Post Hoc Tests For One Way Anova

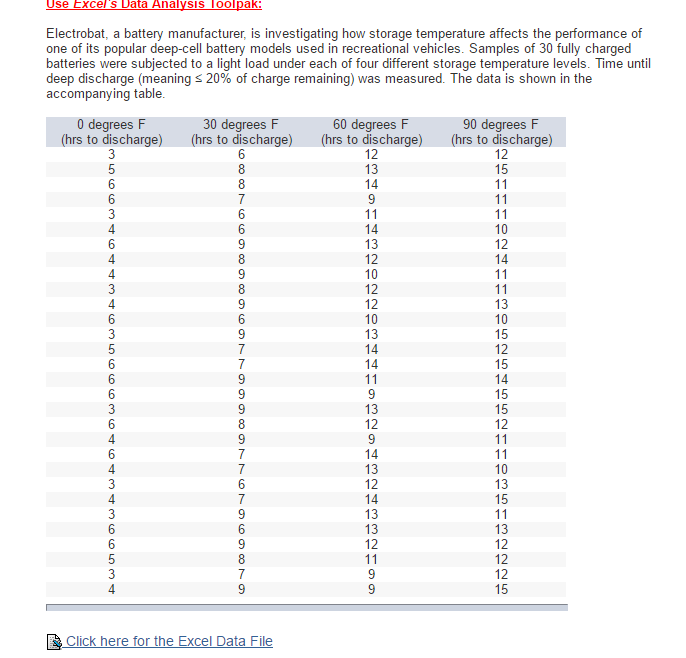
Table 5 Studentized Range Values Q For Tukey S Hsd Chegg Com

Using Post Hoc Tests With Anova Statistics By Jim

Chapter 5 One Way Analysis Of Variance Natural Resources Biometrics

T U K E Y Q T A B L E Zonealarm Results

T U K E Y Q T A B L E Zonealarm Results

Pdf Newman Keuls Test And Tukey Test

Www Sfu Ca Jackd Stat302 Wk04 2 Full Pdf

16 Q Table Statistics

Studentized Range Q Table Real Statistics Using Excel

Post Hoc Definition And Types Of Tests Statistics How To

108 Questions With Answers In Tukey Science Topic

What Are P Values What Are Q Values And Why Are They Important

Anova The Shell Measurement Of 45 Mytilus Trossulus Chegg Com

How Is The Standard Error Used In Calculating A Tukey Hsd Defined And Calculated Cross Validated

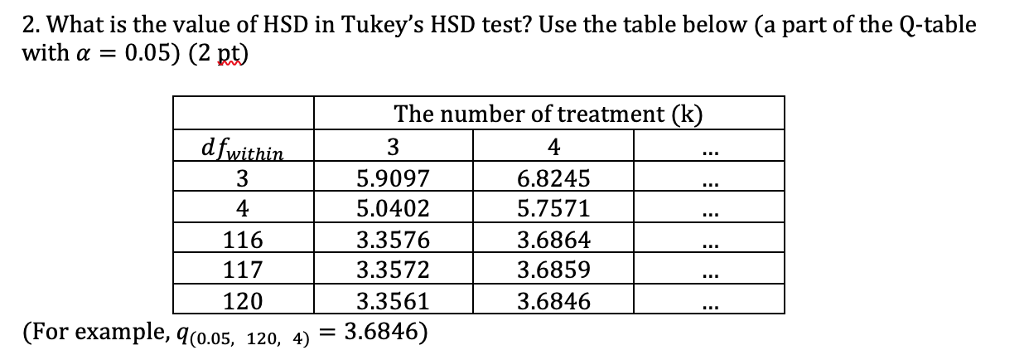
2 What Is The Value Of Hsd In Tukey S Hsd Test U Chegg Com

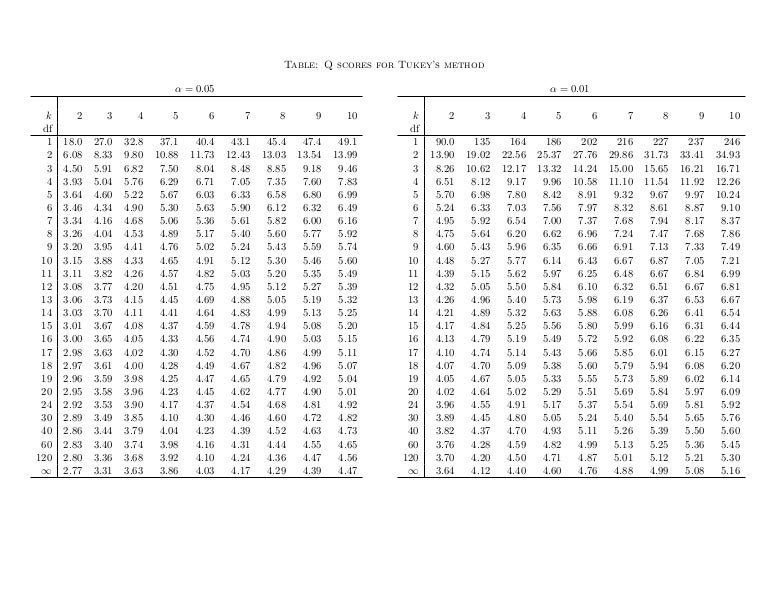
Ex5opofatpt5jm

Q Table Tukey Kramer

A Biologist S Guide To Statistical Thinking And Analysis

43 Q Distribution Table For Tukey Test

Studentized Range Q Table Real Statistics Using Excel

T U K E Y Q T A B L E Zonealarm Results

Post Hoc Tests For One Way Anova

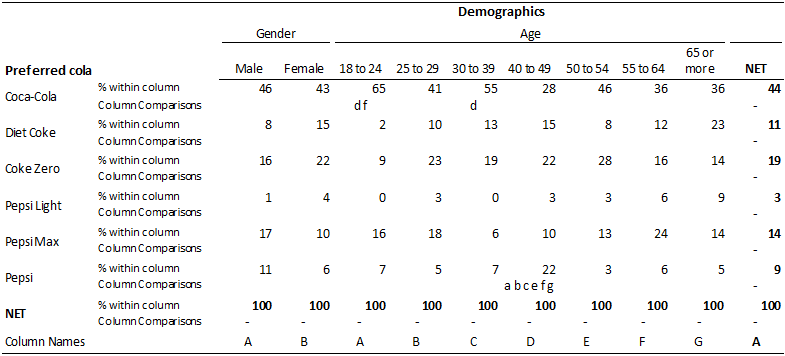
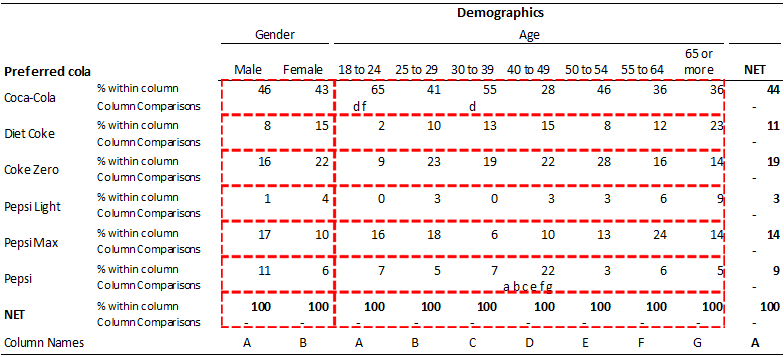
Multiple Comparisons Post Hoc Testing Displayr

Pdf Newman Keuls Test And Tukey Test

Www Jstatsoft Org Article View V085c01 V85c01 Pdf

Ch12 1 Exercises One Way Analysis Of Variance Anova

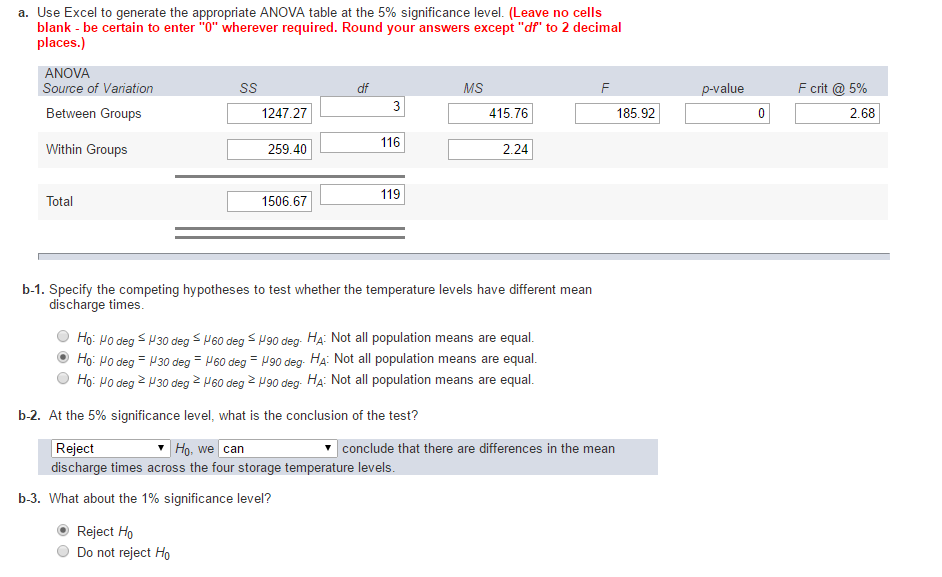
Table 5 Studentized Range Values Q For Tukey S Hsd Chegg Com

T U K E Y Q T A B L E Zonealarm Results

Tukeytable Table Q Scores For Tukeys Method 0 05 K Df 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 24 30 40 60 1 2 18 0 6 08 4 50 3 93 3 64 Course Hero

Turkey Hsd檢驗法 W法 碼上快樂

Post Hoc Tests For One Way Anova

Tukey Hsd Real Statistics Using Excel

How To Calculate The Tukey Kramer And Scheffe P Values

Multiple Comparisons Post Hoc Testing Displayr

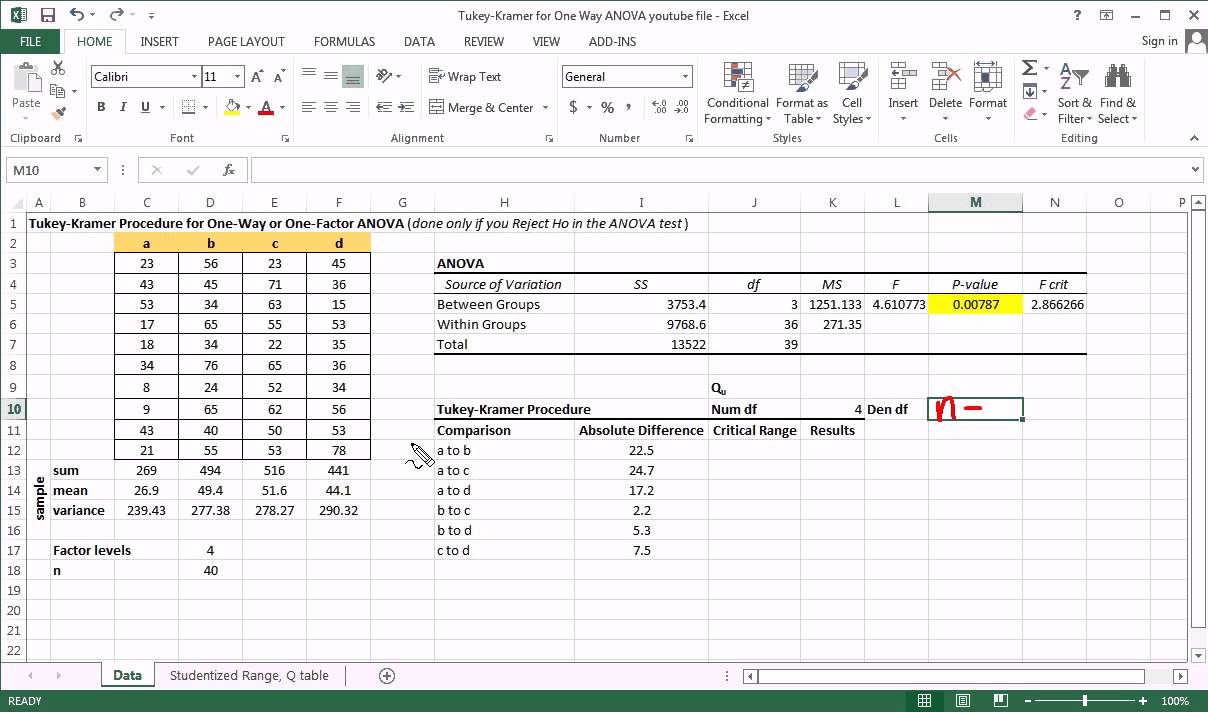
Tukey Kramer Multiple Comparison Procedure And Anova With Excel Youtube

Ijms Free Full Text Udp Glycosyltransferase Genes In The Striped Rice Stem Borer Chilo Suppressalis Walker And Their Contribution To Chlorantraniliprole Resistance Html

Www Ars Usda Gov Arsuserfiles Rapidcalculationofq Pdf

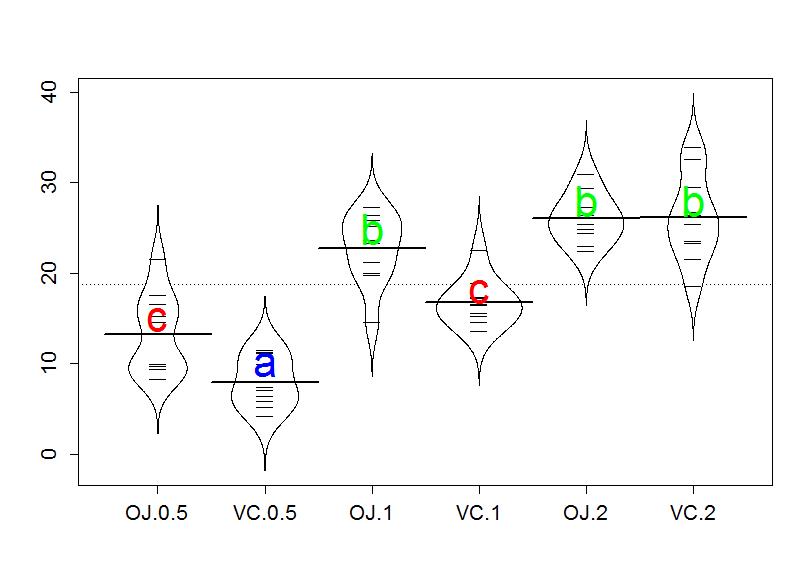
Multiple Pair Wise Comparisons Using Tukey S Hsd And The Compact Letter Display Statistics With R

The Summary Table Of Tukey S Hsd Post Hoc Test Results Among Download Table

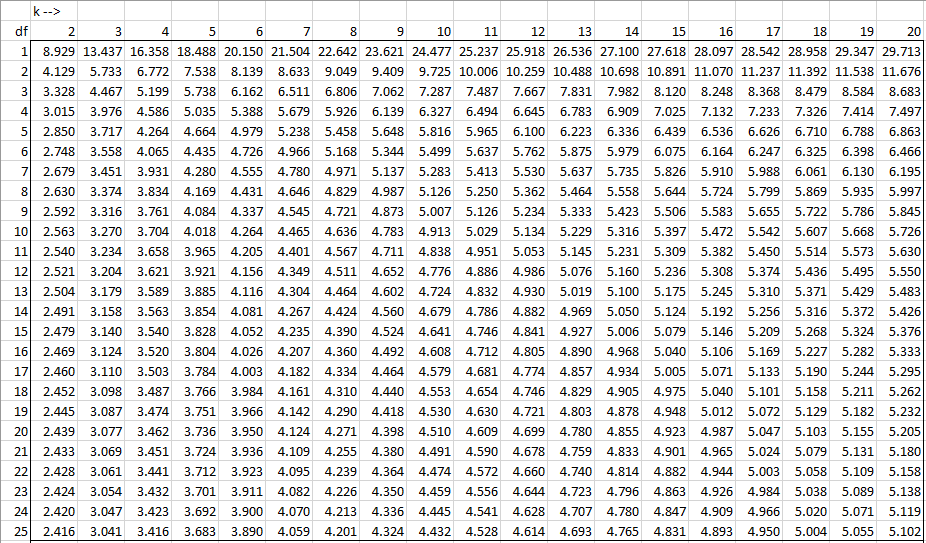
Studentized Range Q Table Real Statistics Using Excel

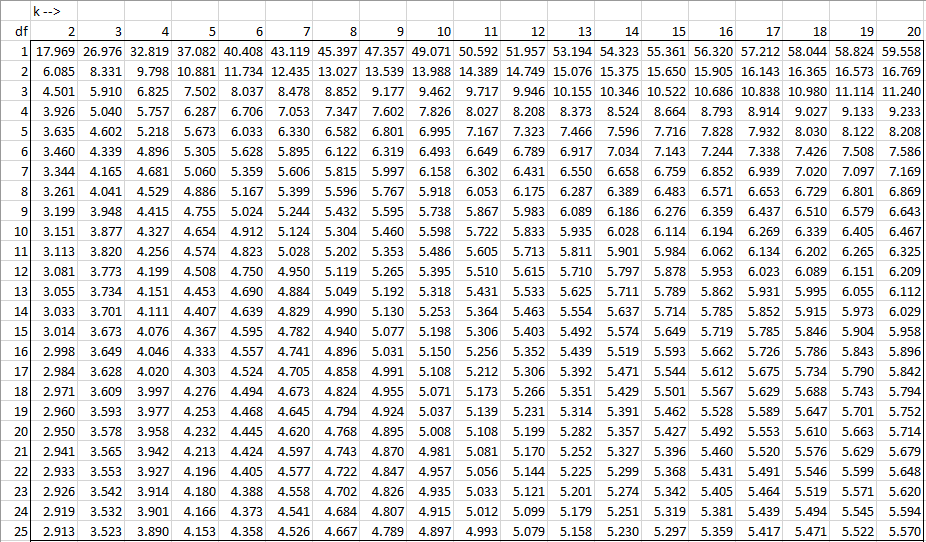
Studentized Range Q Table Real Statistics Using Excel

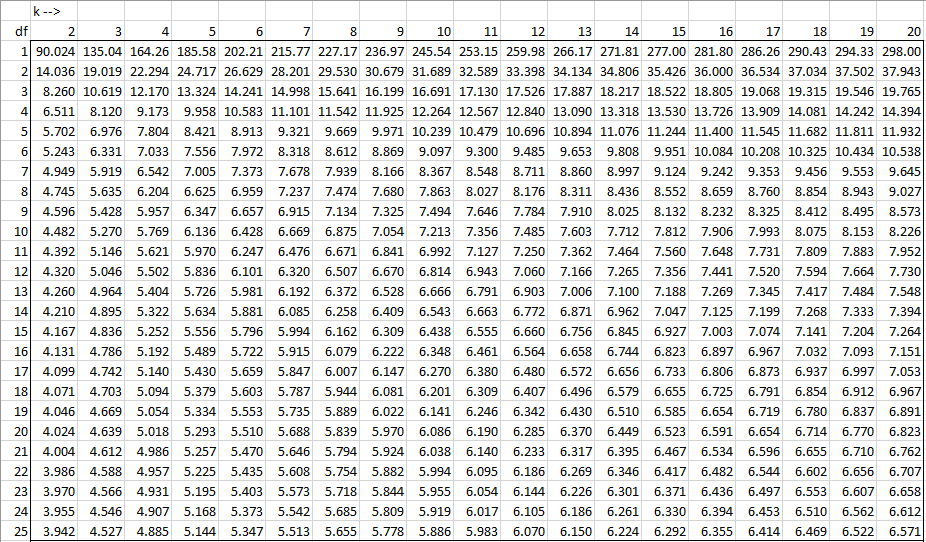
Studentized Range Q Table Real Statistics Using Excel

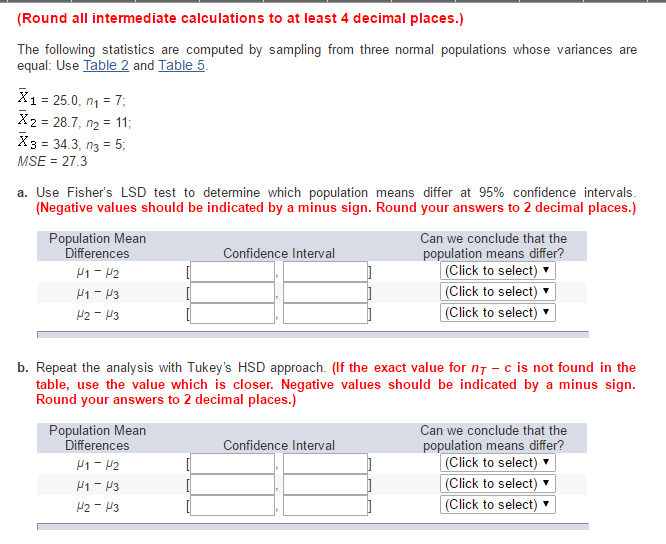
Solved Table 5 Studentized Range Values Q For Tukey S Hsd Chegg Com

43 Q Distribution Table For Tukey Test

108 Questions With Answers In Tukey Science Topic

43 Q Distribution Table For Tukey Test

T U K E Y Q T A B L E Zonealarm Results

Q Table For Tukey Pdf Table B 5 The Q Statistic Tukey Hsd Test K Number Of Treatments Levels Withingroups Df Alp Ha Lev El 2 3 4 5 6 7 8 9 10 11 12 Course Hero

Tukey Pdf 6 Appendix A Tables Table A 9 Upper Percentage Points For The Studentized Range Qvw2 10 11 12 0 10 0 05 0 01 0 10 0 05 0 01 0 10 0 05 0 01 Course Hero

Edit View Insert Format Tools Table Window Help Share Rt Design Layout References Mailings Review 42 Homeworklib

Anova Using Python With Examples